Three famous mathematical formulas
| Prerequisites: | Before attempting this assessment you should have already worked through all the articles in this module, and also have an understanding of HTML basics (study Introduction to HTML). |
|---|---|
| Objective: | To have a play with some MathML and test your new-found knowledge. |
A small math article
The goal is to rewrite the following math article using HTML and MathML:
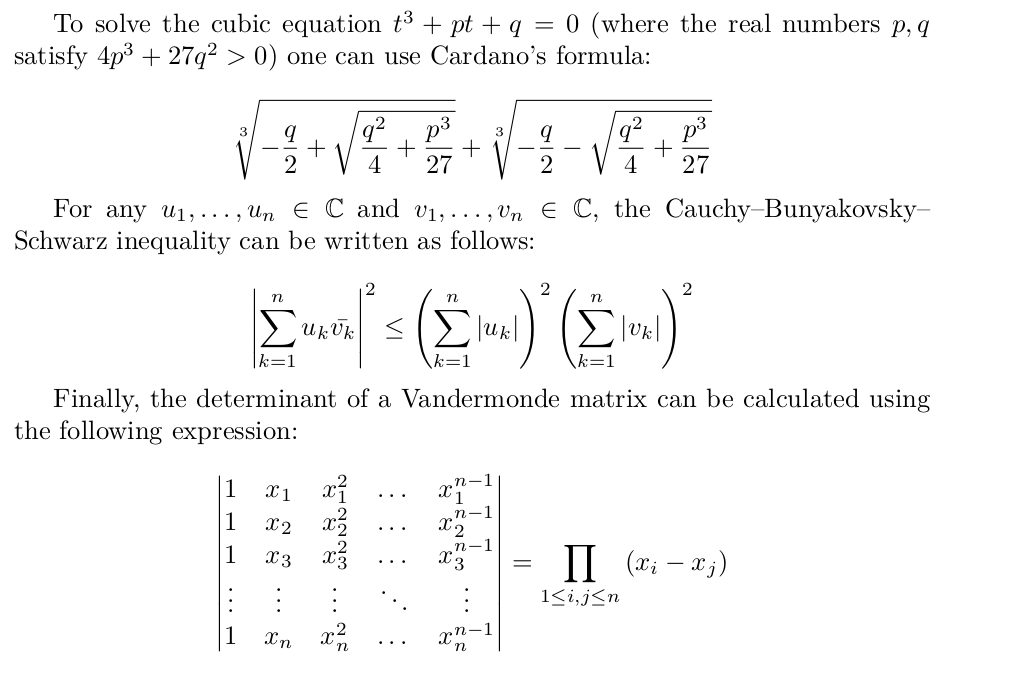
Although you don't need to be familiar with LaTeX, it might be useful to know the LaTeX source from which it was generated:
latex
\documentclass{article}
\usepackage{amsmath}
\usepackage{amssymb}
\begin{document}
To solve the cubic equation $t^3 + pt + q = 0$ (where the real numbers
$p, q$ satisfy ${4p^3 + 27q^2} > 0$) one can use Cardano's formula:
\[
\sqrt[{3}]{
-\frac{q}{2}
+\sqrt{\frac{q^2}{4} + {\frac{p^{3}}{27}}}
}+
\sqrt[{3}]{
-\frac{q}{2}
-\sqrt{\frac{q^2}{4} + {\frac{p^{3}}{27}}}
}
\]
For any $u_1, \dots, u_n \in \mathbb{C}$ and
$v_1, \dots, v_n \in \mathbb{C}$, the Cauchy–Bunyakovsky–Schwarz
inequality can be written as follows:
\[
\left| \sum_{k=1}^n {u_k \bar{v_k}} \right|^2
\leq
{
\left( \sum_{k=1}^n {|u_k|} \right)^2
\left( \sum_{k=1}^n {|v_k|} \right)^2
}
\]
Finally, the determinant of a Vandermonde matrix can be calculated
using the following expression:
\[
\begin{vmatrix}
1 & x_1 & x_1^2 & \dots & x_1^{n-1} \\
1 & x_2 & x_2^2 & \dots & x_2^{n-1} \\
1 & x_3 & x_3^2 & \dots & x_3^{n-1} \\
\vdots & \vdots & \vdots & \ddots & \vdots \\
1 & x_n & x_n^2 & \dots & x_n^{n-1} \\
\end{vmatrix}
= {\prod_{1 \leq {i,j} \leq n} {(x_i - x_j)}}
\]
\end{document}
Starting point
To get this assessment started, you can rely on our usual HTML template. By default it uses UTF-8 encoding, special Web fonts for the <body> and <math> tags (with similar look & feel as the LaTeX output). The goal is to replace the question marks ??? with actual MathML content.
html
<!doctype html>
<html lang="en-US">
<head>
<meta charset="utf-8" />
<title>Three famous mathematical formulas</title>
<link
rel="stylesheet"
href="https://fred-wang.github.io/MathFonts/LatinModern/mathfonts.css" />
</head>
<body class="htmlmathparagraph">
<p>
To solve the cubic equation ??? (where the real numbers ??? satisfy ???)
one can use Cardano's formula: ???
</p>
<p>
For any ??? and ???, the Cauchy–Bunyakovsky–Schwarz inequality can be
written as follows: ???
</p>
<p>
Finally, the determinant of a Vandermonde matrix can be calculated using
the following expression: ???
</p>
</body>
</html>
Hints and tips
- Start by inserting empty
<math>tags, deciding whether they should have adisplay="block"attribute or not. - Check the text used and find their Unicode characters ("−", "ℂ", "∑", ...).
- Analyze the semantics of each portion of text (variable? operator? number?) and determine the proper token element to use for each of them.
- Look for advanced constructions (fractions? roots? scripts? matrices?) and determine the proper MathML element to use for each of them.
- Don't forget to rely on
<mrow>for grouping subexpressions. - Pay attention to stretchy and large operators!
- Use the W3C validator to catch unintended mistakes in your HTML/MathML markup.
- If you are stuck, or realize how painful it is to write MathML by hand, feel free to use tools to help write MathML such as TeXZilla.